这题的描述对我这种语言能力残缺的人来说,复述起来太难:

实质上是求这么一个东西:   G(n)=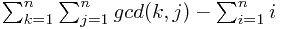

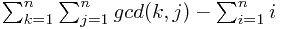
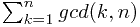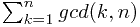求G(n)的话,可以先求T(n)=,然后累加一下...额累加时第i项还要减个i~

如果学过数论,应该有印象,小于n的数里gcd(i,n)=d的i一共有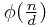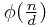个~这意味着:

T(n)=;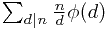

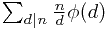现在证明,T(n)是一个积性函数

证明:

设 m , n 满足 (m,n)=1:

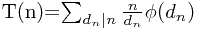
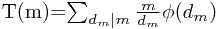
则:

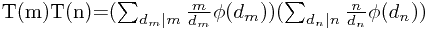
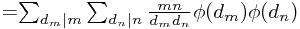
由于(m,n)=1,故dn与dm互素,所有dn,dm的组合正好取遍mn的约数

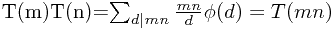
到了这里,只需考虑n等于素数的幂时T(n)的值:

根据T(n)的定义,显然有:

有了这个性质,就有可以在O(n)时间内用这个  牛X的筛法  把表打出来,然后求和还是O(n),查询什么的显然O(1)咯lol...

代码:

#include	<stdlib.h>
#include	<stdio.h>
#include	<string.h>
#include	<math.h>
#define FF(i,b) for(i=0;i<(b);i++)
#define FOR(i,a,b) for(i=(a);i<=(b);i++)
#define ZERO(x) do{memset(x,0,sizeof(x));}while(0)
#define SWAP(a,b) do{int _tmp=a;a=b;b=_tmp;}while(0)
#define INFI 2000000000
#define INFF 1e100
#define maxn 1000000
typedef long long  LL;
int p[maxn+1],ph[maxn+1],pk[maxn+1],T[maxn+1];
LL G[maxn+1];
void sevie()
{
	memset(p,0,sizeof(p));
	int i,j;
	p[0]=ph[0]=0;ph[1]=1;T[1]=1;
	for(i=2;i<=maxn;i++)
	{
		if(!p[i]){p[++p[0]]=i;ph[i]=i-1;pk[i]=i;T[i]=(i<<1)-1;}
		for(j=1;p[j]<=maxn/i;j++)
		{
			p[p[j]*i]=1;
			if(i%p[j]){
				ph[i*p[j]]=ph[i]*p[j];
				pk[i*p[j]]=p[j];
				T[i*p[j]]=T[i]*T[p[j]];
			}
			else{
				ph[i*p[j]]=ph[i]*p[j];
				pk[i*p[j]]=pk[i]*p[j];
				if(i==pk[i])T[i*p[j]]=p[j]*T[i]+ph[i*p[j]];
				else T[i*p[j]]=T[i/pk[i]]*T[pk[i]*p[j]];
				break;
			}
		}
	}
	G[0]=0;
	for(i=1;i<=maxn;i++)G[i]=G[i-1]+T[i]-i;
}
int main ( int argc, char *argv[] )
{
	sevie();
	int n;
	while(scanf("%d",&n)!=EOF)
	{
		if(!n)break;
		printf("%lld\n",G[n]);
	}
	return EXIT_SUCCESS;
}